Monday 27 June 2016
Toomas Karmo: Brexit, Adversity, and Good Cheer
Quality assessment:
On the 5-point scale current in Estonia, and surely in nearby nations, and familiar to observers of the academic arrangements of the late, unlamented, Union of Soviet Socialist Republics (applying the easy and lax standards Kmo deploys in his grubby imaginary "Aleksandr Stepanovitsh Popovi nimeline sangarliku raadio instituut" (the "Alexandr Stepanovitch Popov Institute of Heroic Radio") and his grubby imaginary "Nikolai Ivanovitsh Lobatshevski nimeline sotsalitsliku matemaatika instituut" (the "Nicolai Ivanovich Lobachevsky Institute of Socialist Mathematics") - where, on the lax and easy grading philosophy of the twin Institutes, 1/5 is "epic fail", 2/5 is "failure not so disastrous as to be epic", 3'5 is "mediocre pass", 4.5 is "good", and 5/5 is "excellent"): 4/5. Justification: There was enough time to develop the relevant points to reasonable length.
Revision history:
- UTC=20160628T2018Z/version 1.2.0: Kmo made a substantive revision on the basis of fresh e-mail from a London friend, amplifying the discussion of the "350 million GBP per week" in the pro-Brexit leaflet, and calling on readers to help him get further particulars (and he revised the right to make also tiny, nonsubstantive, revisions, without formal documentation in this revision history, over the coming 30 days, as versions 1.2.1, 1.2.2, 1.2.3, ... ).
- UTC=20160628T1545Z/version 1.1.0: Kmo made a substantive revision, improving his discussion of the GBP-versus-USD exchange-rate history (and he reserved the right over the coming weak to make tiny, nonsubstantive, revisions, without formal documentation in this revision history, as versions 1.1.1, 1.1.2, 1.1.3, ...).
- UTC=20160628T1526Z/version 1.0.8: Kmo made further tiny tweaks, which he deemed sufficient to justify a "patch" incremenent from 0 to 8 in the "major.minor.patch" formalism; and he reserved the right over the coming week to make further tiny, patch-level, tweaks, without formal documentation in this revision history, as versions 1.0.9, 1.0.10, 1.0.11, ... .
- UTC=20160628T0001Z/version 1.0.0: Kmo uploaded base version (and planned to upload in the ensuing four-hour interval, without formal documentation in this revision history, nonsubstantive revisions, as versions 1.0.1, 1.0.2, 1.0.3, ...) .
0. Preamble
2016-06-14 was the publication day on this blog for my reticent note "Contribution to Brexit Debate (Diplomatic-Leverage Question)". As an Estonian national currently living in Canada under dual Estonian-Canadian citizenship, and as a resident in Britain from 1974 September through 1978 August, I felt I had to say something on 2016-06-14, even while keeping my contribution terse.
But on writing back then, I had failed to imagine the potential full gravity of the looming situation. Now, with the actualization of what was already then imaginable by the pessimist, I do best to write at length.
1. Situation Assessment: A USSR-1991 Rerun?
In that 2016-06-14 note, I pointed out the need to cast the Brexit debate in terms deeper than the crassly economic.
To be sure, economics has its (modest) place. We may as well start there, before advancing from pounds and pence to things that more deeply matter.
Most of us - in Canada, in the UK, in Estonia, in the USA, almost anywhere - have run out of patience with our current business leaders. We see only too plainly how our towns here in Ontario and Nova Scotia have in the last quarter-century been hollowed out, to the point where our once-vibrant main streets have been relegated to the twenty-dollar psychics, to the porn shops, and to the Golden Arches. Here in Richmond Hill, even suburban malls are now showing signs of failure - as when Timothy's is replaced by Starbucks, and Starbucks is in turn replaced with brown paper behind silent glass.
We see how difficult it is now for young people to establish careers in growing things or making things, as opposed to establishing their careers in the mere trafficking of symbols. And even the latter type of career is far from what it was twenty years ago. Tech support, I gather, is still a career option in Ontario I.T. But I have the concomitant impression that much of the Java or Python coding - the duly skilled work - has gone offshore.
We see, if not from direct experience then at least from reading, how drastic these developments have been in that Mother of All Rustbelts which is the formerly industrialized Britain. To take one example: shipbuilding in Britain has been comatose for decades, with the small twin exceptions of naval work (now gone?) and rich-guy yachts. Or, to take another example, we find in Mike Carter's 2016-06-27 Guardian opinion piece "I walked from Liverpool to London. Brexit was no surprise", at https://www.theguardian.com/commentisfree/2016/jun/27/liverpool-london-brexit-leave-eu-referendum, the following description of Walsall: "Everywhere there were betting shops, dozens of them, and right next
door to every betting shop was a pawnbroker or payday lender. It was a
ghoulish form of mutualism, or symbiosis, the 'natural' market at its
most efficient." And from the same journalist comes an impression of Nuneaton: "/.../ more charity shops in its high street than anywhere I've ever seen. And
some of those charity shops had closed down. What does it say about a
town when even the charity shops are struggling?"
Yet the people who deal professionally in money have so far proven smart enough to get ahead.
Which way have those smart operators been betting in recent days? One thing they do not seem to have a lot of confidence in is the pound sterling. The decline in GBP against USD on the first major trading day, 2016-06-24 (FRI), after the referendum was the sharpest in at least decades. Although we must eventually see some kind of modest rally in sterling, the decline has at any rate continued on my main day for writing this essay, 2016-06-27 (MON). At UTC=20160627T190509Z, my screen is showing one GBP to be commanding a meagre 1.32 USD. In 2016 May, the rate, as taken in a daily timeframe by http://www.investing.com/currencies/gbp-usd-historical-data, had been rather higher and rather stable, varying between the extremes of 1.4673 and 1.4401.
****
Turning now from economics to deeper points of governance, we note first that the referendum has triggered a meltdown in the two dominant UK parties. It is a meltdown with minimal, or with no, UK precedent. The Conservatives now face a leadership contest. Since, moreover, Mr Cameron's government has not as of this writing taken a decision on the EU's Article 50 (the referendum does not legally bind him), it is beginning to appear as though he may be intending to hand his - as yet unknown - successor a poisoned chalice, with eventual further harsh consequences for the Conservative party. (For the destabilizing poisoned-chalice scenario, on which it is Mr Cameron's successor, rather than Mr Cameron, that gets to launch Brexit by formally invoking Article 50, I would refer the reader to an excellent blog by legal scholar David Allen Green, at http://jackofkent.com.) Labour, on the other hand, has lost a row of Shadow Cabinet figures, with a leadership vote-of-no-confidence an emerging possibility. It is the extremists, outside the traditional ranks of Conservatives and Labour, who are most likely to benefit from the Westminster tumult.
Second, we note the agitated state of Scottish public opinion. Every Scottish voting district sided with "Remain". (On such regional questions, the maps and tables at http://www.bbc.com/news/uk-politics-36616028 are particularly helpful.) Some are now complaining that Scotland will be dragged out of the EU against its will. Some additionally infer from this emerging complaint the emerging advisability of a 2018 Scottish "IndyRef2".
Third, we note the likelihood, over coming months, of a resonant agitation in Northern Ireland. Northern Ireland, like Scotland, had an overall majority voting for "Remain" - even though in that jurisdiction, in contrast with Scotland, some local voting districts did favour "Leave". Many members of the Catholic minority in Northern Ireland, already before 2016-06-23 rather willing to entertain the idea of reuniting Northern Ireland with EU member state Eire, will now be still more willing. The Protestant majority in Northern Ireland, historically cool to Irish reunification, in large measure comprises descendants from 17th-century "Ulster Scots" immigrants. This segment in the community might now for its part take some special interest in the evolving Scottish debate - taking due note of Scotland's strongly pro-EU, and in that context separatist, inclinations.
These three points suggest a scenario on which the UK shrinks over the coming decade into a mere "United Kingdom of England and Wales" - a little before a rising North Sea starts washing over the East Anglian fenlands, and over London around the Embankment.
This conceivable drama looms in the context, moreover, of seldom-seen electoral tension in the USA, as the (in my view) unacceptable candidate Trump squares off against the (in my view) unacceptable candidate Clinton. Mr Trump seems a demagogue, willing to countenance even violence at his rallies. Ms Clinton's EmailGate troubles, some are arguing, will intensify, with the FBI a legal factor. I believe, without having at present done more than glance superficially, that https://20committee.com probes this side of the electoral contest, considering the loudest legal drama to lie as yet ahead of us.
So now we have a worst-case scenario, against which we must try to brace ourselves emotionally. Perhaps, on this scenario, 2016 will go down in history as the year the UK-USA Anglosphere started to break, with normal political life disintegrating in both countries, in an upheaval of ultimately Gorbachevian proportions.
2. "Remain" Counterarguments to a Pro-Brexit Flier
Just after the referendum, a friend in London, in one of the NW postcodes, kindly e-mailed me a PDF scan from a one-sheet flier. The flyer had been delivered to his place of residence by the "Leave" side. It is advisable that in this essay I spell out, with details, my case for "Remain". Although the referendum is over, the real crisis is starting, and all of us everywhere will need to keep our eyes open.
An easy way to discharge my duty of clarification is to counterargue, against the flier, so to speak clause by clause. Helpfully, the flier lays its advocacy out with short bullet points. In each case, the flier prints the core of its point in bold type, adding a little bit of amplification in lightface. I will repeat this style of typography, but with my own successive commentaries - in essence, rebuttals - set in italics. The one special emphasis (I use underlining for it) is in the original:
- Over a quarter of a million people migrate to the UK from the EU every year. This is the equivalent of a city the size of Newcastle every year. EU law means all members must accept 'the free movement of people'. Many immigrants contribute to our society. They also have an impact on public services. Experts disagree on the overall effect. - No mention is made of the fact that the UK also takes in large numbers of immigrants from outside the EU. Further, the 'many immigrants' contribute' is a backhanded way of saying, rudely, in the manner of a modern Enoch Powell, 'Some do not.' Apart from being veiled demagoguery, this is not fully logical. The aged, the infirm, the weak, and the demoralized or criminal UK native-born could with equal justice (or with equally cruel injustice) be castigated as 'not contributing'.
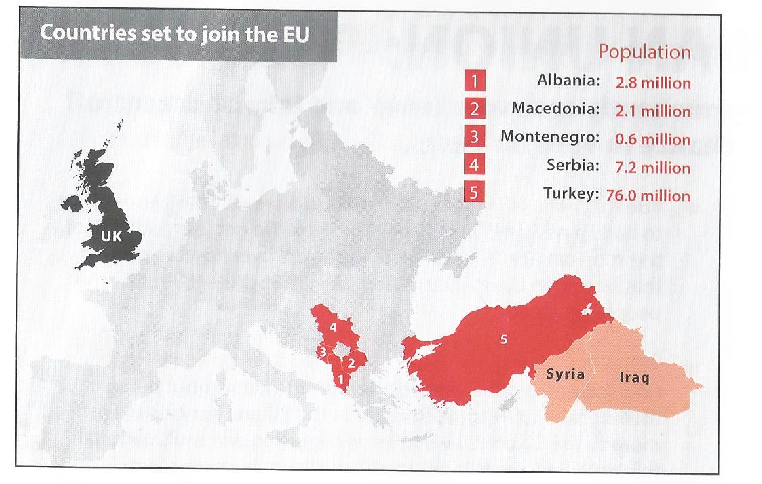
- The EU is expanding to include: Albania, Macedonia, Montenegro, Serbia, and Turkey. When we joined, there were just 9 members states. Now there are 28, the most recent being Romania, Bulgaria and Croatia. Five more contries are in the queue to join, totalling 89 million people. When they join, they will have the same rights as other member states. - It is left unexplained that according to the 2016 Turkish census, Turkey's population is to three significant figures 79.5 million (in other words, that the overwhelming majority of the "89 million" are Turkish); that Turkey has been in EU accession negotiations since 2005; that EU membership for Turkey can only be granted if Turkey clears high ethical hurdles regarding human rights, and the like, as the existing EU members have, through devising and conducting some EU-accepted process of domestic reform; and (this is philosophically crucial) that if Turkey were to clear the ethical hurdles, then Turkey would have become as ethically respectable a member of the global community as the existing EU members are. At that future point, if it is reached, the objections to Turkey's joining could not be anchored in any coherent stance in political philosophy, but only in prejudice.
- The European Union has changed enormously since the UK joined the 'Common Market' in 1973. The EU has taken control over more and more areas such as our borders, our public services, and VAT. The need to prop up the Euro means that more and more powers will be taken by the EU. - This, although vague, is the best of the various bullet points. It is true that the EU has "taken control" in many areas, and it may be that the control is in various cases excessive. I do, however, counterargue that control was taken upon discussions among the member states (the UK included), with due engagement both by national parliaments and by the European Parliament, and that controls once imposed can in later deliberations be reformed, at any rate given a democratic consensus among the disadvantaged people. - Further, I counterargue that the UK, as a big country, cannot plausibly complain of being steamrollered. If you are small, like Estonia, you might in theory be somewhat vulnerable to steamrollering. If you are big, like the UK (historically not always even polite in Brussels), you are, on the other hand, less vulnerable. - Finally, I counterargue that the UK will in any case now be "controlled" by a Continent that can threaten to impose unfavourable terms of trade, or even to impose some border formalities. There is plenty of "control" that can get imposed on you in diplomacy, especially once you have distanced yourself from your former partners - in other words, once you have given your former partners excuses for throwing hard balls at you.
- EU law overrules UK law. This stops the British public from being able to vote out the politicians who make our laws. EU judges have already overruled British laws on issues like counter-terrorism powers, immigration, VAT, and prisoner voting. The new 'deal' David Cameron negotiated recently can be overturned by the European Court after our referendum. - This argument cuts both ways. While the EU has reduced (it has not struck down) the legislative powers of Westminster, the EU also provides the UK subject some recourse against Westminster excesses. Wait, I remark to the prospective Brixiteer, until Westminster elects a government you really, really dislike (this historically happens every five or ten or fifteen years, no matter what your political inclination might be, since in the the UK Left and Right historically shuttle back and forth): at this point, you may long for a Westminster-Brussels duo to provide "checks and balances". - I find this very phrasing, of checks and balances, used independently of me, in a helpful blog comment by reader "luna" at John Michael Greer's http://thearchdruidreport.blogspot.com, timestamped by Mr Greer's server as "6/27/16, 2:04 AM".
- The EU costs us at least £350 million a week. That's enough to build a new NHS hospital every week. We get less than half of this money back, and we have no control over how it's spent: that's decided by politicians and officials in Brussels, not by the people we elect. - So that's about 5.50 GBP per head of UK population per week laid out, with something (admittedly, "less than half") eventually even coming back. The sum of 5.50 GBP per week, in comparison with what people have to fork out every week in total, in VAT (the UK sales tax), and as their weekly portion of their annual property taxes and annual income tax, is not too intimidating taxwise, even if (contrary to what is the case) none of it comes back - particularly given that this sum is being used to try to get, among other things, good terms of global trade for the EU, the UK included. - (1) I have found from my own quick Web researches some controversy, favouring "Remain", surrounding the 350 million GBP claim which underlies my little 5.50 GPB-per-capita calculation. Additionally, (2) I am told on 2016-06-28 by my London friend, in continuing e-mail correspondence, that according to The Economist (the weekly newsmagazine) the correct gross figure (i.e., the figure before we net off the "less than half" that eventually comes back) is itself significantly below the 350 million GBP stated here. My friend thinks, from memory, that The Economist gave as the correct gross figure, in place of 350 million GBP, 250 million GBP. (My friend also remarks that The Economist noted the further reduction which ensues when we go net, not gross: down to something, he thinks, around one-third of 350 million GBP.) If some reader can help me here - e-mail, please, to Toomas(dot)Karmo(at)gmail(dot)com, optimally with quote from The Economist, plus date of publication and page number - I will revise this essay accordingly, updating its version number in my top-of-essay revision history.. - I will comment more about terms-of-trade under the next two bullet points. For the moment, however, I note here a feature of political discourse surely pertinent to the "Remain" side of the debate just as much as to the "Leave" side, and in fact pertinent to every political debate I have ever seen, in any country at all. Please, folks (pols, editorialists, television writers, bloggers, you whole sorry lot): don't express weekly exchequer outlays in gross millions without also putting your numbers into context, by expressing them in parentheses as per-capita weekly exchequer outlays.
- You don't have to be a member of the EU to trade with it. Countries across the world trade with the EU without being members of it. Switzerland is not in the EU and exports more to the EU than we do. Some big banks and multinationals think the EU is in their interests. Small and mediuim-sized business think differently. Only 6 per cent of UK firms export to the EU, yet all have to obey EU rules. - If you as a state are not yet a member of the EU, it is, other things being equal, a little harder for you to trade with the EU than it is for an EU member to trade with the EU. You need to negotiate some special arrangement, as Norway has, and as Canada is now trying to. The special arrangement has, at least in the case of Norway, involved making contributions to the EU budget, similar in size to the contributions made by the EU member states themselves. There is indeed nothing to stop the EU trade negotiators from playing diplomatic hard ball over such things as your own domestic refugee-intake policy, even outside the strict sphere of economics (cf bullet point on "control", above).
- While we're in the EU, the UK isn't allowed to negotiate our own trade deals. This means we currently have no trade deal with key allies such as Australia, New Zealand, or the USA - or important growing economies like India, China or Brazil. Instead of making a deal which is best for the UK, we have to wait for 27 other countries to agree to it. Most small businesses say that Britain should take back the power to negotiate our own trade deals which we cannot do inside the EU. - This argument cuts both ways. It is also true that the EU has greater negotiating power in global markets than the UK would if negotiating solo.
- There are risks in voting either way. Experts, politicians, and businesses are divided. People have to weigh up the risks and potential benefits of each course of action for themselves. - Agreed.
3. Has Public Opinion Been Manipulated?
There is a line which I conjecture must never be crossed in court. This conjecture I make as a non-lawyer, but all the same on the strength (because of my work in natural-heritage and cultural-heritage conservation) on the strength of some minor presence at proceedings.
Advocacy in the courtroom, even to the point of omitting facts injurious to your side and beneficial to your adversary, is allowed. I have never to my knowledge done it personally, and I hope never to be intimidated into doing it personally - whether in the context of the David Dunlap Observatory urban-forest conservation battle or in any other context, in any country. I would, however, have to forgive it if it got done to me.
It cannot, on the other hand, be excusable in the courtroom to outright mislead - to show a graph, for instance, which misrepresents a trend by resorting to an unexpected, and visually understating, logarithmic scale on your vertical axis, while in showing it hoping that nobody will notice how you chose your scale.
To my surprise and alarm - I did not think UK politicians would sink to these depths - the "Leave" flier has as its principal graphic a misleading map (available on this blog in a separate posting). Albania, Macedonia, Turkey, Montenegro, Serbia, and Turkey are coloured red, with accompanying text to the effect that they are candidates for EU membership. The map additionally shows two states, Syria and Iraq, in orange, without explanation in the accompanying text. The plain, albeit unwritten, suggestion is that Syria and Iraq are somehow states relevant to the topic of EU enlargement. Indeed the incautious reader is invited, by the context although not by the explicit words, to infer that Syria and Iraq stand in an accession queue behind the five states coloured red.
The rest of the map is in shades of grey. The grey, however, is not uniform. The grey is quite dark over the three Baltics, Byelorussia, and Ukraine. Here the visual suggestion is, contrary to legal fact, that Byelorussia and Ukraine (not in the EU, and not even close prospects for EU accession) are in the same EU treaty position as the three Baltics (who have been in the EU for years).
The shading is lighter over western continental Europe, with Portugal virtually disappearing on my screen. Spain and Italy are duly visible.
The oddest thing of all is that while Northern Ireland is duly visible, Eire disappears altogether, at least on my screen - and so, I presume, if not on all screens, at least on the screens of any who share my particular level of poverty, and who in consequence have rather old and rather downmarket flat-screen monitors (in my case, the modest old LG Flatron W1952TQ). Was the artist trying to make voters overlook the circumstances of EU member Eire - perhaps aware that many in London, while not themselves Irish, will all the same have over in Eire cousins-of-girlfriends, pals-of-cousins, and the like?
4. Can we "Keep Calm and Carry On"?
It is essential in public affairs to avoid what I will here call the "Optimist's Fallacy". The political Optimist is one who thinks that when things are bad, a shakeup, as opposed to a piecemeal reform, is liable to improve them.
Sometimes shakeups do help. The already-cited 1991 end of the USSR, under M.S.Gorbachev, brought a swift improvement in the human-rights, and an eventual improvement in the economic, conditions of northeastern Europe. The rapid 1947 end of the Raj might, for all I know, have been the best available thing for India (although here it might contrariwise be asked whether some kind of slower, say five-year, winding down could have prevented or reduced the terrible regional war over Partition).
As often as not, however, shakeups disappoint.
Cromwell worked out, on the whole, badly.
Edmund Burke, celebrated for his caution, rightly denounced the French Revolution. It is true that he applauded the American Revolution. But was he right in applauding it? Had the Thirteen Colonies resolved their grievances with Westminster through mere political agitation (this was, with just minor exceptions, the path taken in the 1830s by Upper Canada, Lower Canada, and Nova Scotia), slavery might have ended in the South under Westminster's 1833 Slavery Abolition Act, as it ended in British North America. In that case, the American Civil War might have been avoided. So, I respectfully suggest, "Mr Burke, you were clearly right on France, and yet possibly wrong on America."
This Brexit thing might work out, and it might not work out. It was in any case reckless of Mr Cameron to gamble, for what were ultimately reasons internal to the Conservatives and their farther-Right UKIP challengers, on momentous stakes.
****
When under existential threat in the Hitler war, the UK Government produced a series of big posters, the last of which was famously headed "Keep Calm and Carry On". The "Keep Calm" press run comprised 2.45 million copies - enough, in other words, to allow one such poster for every (to two significant figures) eighteen wartime UK residents.
Things got bad in the Hitler war. Although the real danger lay in a possible negotiated capitulation, rather than in an invasion (an amphibious assault requires preparations, considered by many or most 21st-century analysts to be beyond the scope of the Reich's hasty 1940 Unternehmen Seelöwe river-barge improvisations), contingency plans were nevertheless drawn up for evacuating the Royals to Canada. A small chain of safe houses was selected, at a few points progressively closer to the Liverpool docks. Plans were also made for broadcasts by the King or other authorities, as the Government made its envisaged orderly westward retreat.
As things turned out, the "Carry On" posters were never needed. They did not resurface until modern times. Now we have wonderful knockoffs, such as "Keep Calm and Knit On," and "Keep Calm and Learn Latin." I for my part want someday to put up, here and there in the University of Toronto, "Keep Calm and Carry on Promoting a Formalism for Multivariate Calculus in which the Leibniz 'd' and 'curly-d' are Suppressed, with Due Recourse Made Instead to the Alonzo Church Lambda-Abstraction Notation in the Spirit of Sussman-Wisdom's Structure and Interpretation of Classical Mechanics."
How do we, in the current situation, with the UK possibly headed for economic diminution, and possibly also for a territorial diminution, keep calm?
It helps to think of this UK crisis as a potentially bracing move to a new, and manifestly inferior, neighbourhood.
Groceries used to be readily affordable. Now, in these unfamiliar new shops, they cost more - perhaps reflecting the ratio (in light of this month's movements in GBP against USD) between 1.47 of something useful and a mere 1.32 of that same useful article.
It used to be that UK bosses were reined in by some distant Continental laws, protecting safety in the workplace, and that UK constables had to be careful to avoid Continental court challenges. Now the bosses and their natural allies, the constables, get a freer hand.
But will the English and Welsh, in this conceivable "Amazing, Shrinking, UK", be downhearted, as their economy contracts and the warming North Sea rises? Perhaps everyone will now be exploring what special things their new neighbourhood might have to offer. Life goes on, and in every bad situation some good can be found.
Such an attitude is not optimism, such as the Parisian poor inappropriately took in 1789. It is, rather, a cheerful pessimism.
Good cheer can thrive in adversity. Adversity is a condition to which people in England and Wales (as, indeed, in other interesting jurisdictions, Estonia among them) are already long accustomed.
[Next week, if the UK crisis allows it, I should again be blogging on the "Is Science Doomed?" theme.]
Tuesday 21 June 2016
Fred Hoyle: inspirational extract on methods-of-study, from his collection "Encounter with the Future"
[The following material is copied verbatim (the italics are in the original) from the essay entitled "Reflections and Reminiscences", in an essay collection by the late Prof. Fred Hoyle, published in book form under the general title Encounter with the Future (New York: Simon and Schuster, 1968). This particular excerpt spans pages 81, 82, and 83. It is quoted here as an authoritative broad corroboration - Hoyle had authority as one of the leading twentieth-century astrophysicists - of the position I broadly take in "Part D" of my own earnest-if-minor essay "Is Science Doomed?". I must not exaggerate by suggesting, without qualification, that Prof. Hoyle's passage is (a) a corroboration of my specific argument against a neomediaeval, neoscholastic, deference to scientific authority, and contrariwise (b) a corroboration of my specific argument for acquiring a grasp of the subtler mathematical side of scientific method through independent labour on conceptually deep questions. (My own example, in "Part D", is a sequence of questions anchored in "Faraday's Paradox" or "Faraday's Puzzle", seeming at a superficial level to refute a Maxwell equation.) The exaggerated phrasing is what I had used in my comment on this page up to UTC=20160622T1725Z. All the same, Prof. Hoyle explains things along the same broad and general lines as I do, stressing the importance of discovering the maths for oneself. I do speculate that if he were alive to be queried, he might prove willing develop his here-quoted ideas further, addressing my own distinction between the first two-thirds of the scientific terrain (for which, I argue in "Part D", routine problem-set assignments suffice) and the mission-critical final third (for which, I argue in "Part D", we have to attempt something like original work, even when within the confines of the classics). - I assume here, subject to correction by Simon and Schuster or their assignees, that my excerpt, being a short extract from Prof. Hoyle's long essay, satisfies the "fair use" provisions of copyright law.]
/.../ where a child is keen to learn, present methods seem woefully and even shockingly inadequate. For the keener student an absolute minimum of formal teaching should be prescribed. I will take mathematics as an example, because mathematics is supposed to be the hardest of all subjects to learn. I suspect it would be possible, given sufficient incentive, to design a complete set of examples, starting with the first or second grade, and ending at a stiff university standard. Perhaps a hundred thousand examples would be needed to cover the whole range. The correct procedure I am sure is to learn by doing, not by being told what to do. /.../
/.../ The need is for someone clever enough to build our best textbooks into an appropriate set of problems. Would children work under these conditions? To me at least, working a problem successfully, looking at the answer and checking that I am right, has always been vastly more interesting than formal lessons. I suspect this is a natural human reaction, and that the same is true for everybody. The reason why mathematics is too difficult for the majority of people is that the examples given to the student at the end of a class are too complicated, unless what has been said in the class has been thoroughly understood. Since the majority of students do not, and cannot, understand by hearing instead of doing, the normal experience is to find oneself completely incapable of solving the required exercises. This would have been obviated if the first exercises had been extremely easy and had been followed by slightly less easy ones, and so on.
All these things apply a fortiori at university level. /.../
Toomas Karmo (Part D): Is Science Doomed?
Quality assessment:
On the
5-point scale current in Estonia, and surely in nearby nations, and
familiar to observers of the academic arrangements of the late,
unlamented, Union of Soviet Socialist Republics (applying the easy and
lax standards Kmo deploys in his grubby imaginary "Aleksandr
Stepanovitsh Popovi nimeline sangarliku raadio instituut" (the
"Alexandr Stepanovitch Popov Institute of Heroic Radio") and his grubby
imaginary "Nikolai Ivanovitsh Lobatshevski nimeline sotsalitsliku
matemaatika instituut" (the "Nicolai Ivanovich Lobachevsky Institute of
Socialist Mathematics") - where, on the lax and easy grading philosophy
of the twin Institutes, 1/5 is "epic fail", 2/5 is "failure not so
disastrous as to be epic", 3'5 is "mediocre pass", 4.5 is "good", and
5/5 is "excellent"): 4/5. Justification: There was enough time to
develop the relevant points to reasonable length.
Revision history:
- UTC=20160622T1709Z/version 1.1.0: Kmo made a string of substantive improvements (remarking on the need to incorporate nuclear-physics assumptions into the mathematical modelling of the solar interior; and anticipating an undergraduate objection to a superficial exposition of Hume; and adding a further example (the Feynman oil slick), which he had this week forgotten, from his recapitulation here of his writing on Mr Greer's circa-2016-02-17 blog; and adding to his discussion of the the Earth mass determination the need to measure gravitational acceleration of falling bodies, as one of the inputs to the eventual mass calculation; and making his ideas about scholastic ossification clearer through a simile or metaphor of terrain-only-two-thirds-covered). He retained the right to make minor, nonsubstantive, improvements over the coming days, without formal documentation in this revision history, as versions 1.1.1, 1.1.2, ... .
- UTC=20160621T0001Z/version 1.0.0: Kmo uploaded base version (and planned to upload in the ensuing four-hour
interval, without formal documentation in this revision history,
nonsubstantive revisions, as versions 1.0.1, 1.0.2, ...) .
****
We can now see where conservation priorities must lie as we determine, so
to speak, how far behind the breached Mannerheim Line our forces shall
retreat.
It was strongly argued by John Michael Greer at http://thearchdruidreport.blogspot.com, I think at some point in 2014 or 2015, that a priority in our impending Dark Age must be the conservation of a grasp of scientific method, as opposed to a mere conservation of factual knowledge. To this I assent, with amplification and development.
I have suggested that within the field of method, it is of primary importance to preserve a grasp of those aspects of method that pertain to the enunciation of laws, as opposed to those aspects that pertain to the discovery of manifest contingencies.
We sometimes encounter thin discussions of the "scientific method", in the schema "Make observations, formulate hypotheses, deduce testable predictions, and perform the tests." Many of us will remember sitting through such a sermon in those Places of Scant Learning which are our North American high schools.
The scheme fits some areas of work well enough. It fits double-blind drug trials, as at Servier or SmithGlaxoKline. And (a happier example) it would usefully fit an investigation into the efficacy of mycorrhizal associations - nowadays of agronomic interest as a biosphere-friendly alternative to phosphate fertilizers.
The scheme is, however, of only limited use in guiding the investigation of fundamental principles. The core of the scientific method where it really matters, at the level of principles rather than of individual facts, is hard to capture in a slogan or schema. There is no obvious sense in which Maxwell, in deciding that the line integral, all the way around a non-self-intersecting loop, of magnetic field, had to be sensitive to the rate of time variation in the integrated "Electric Illumination" of the various capping surfaces, was proceeding from "observations". (It was, rather, the correlative principle, that the line integral of electric field is sensitive to the rate of time variation in the integrated "Magnetic Illumination" of the loop's various capping surfaces, that was the well-known observation-backed hypothesis.) The core is, on the other hand, readily demonstrated with examples.
If a slogan or schema is all the same demanded, the following will serve in its bare-bones fashion, given an abundance of corroborating examples: "Mathematics has explanatory power in the physical world."
****
The very idea of mathematics as possessing explanatory power is somewhat resisted in contemporary culture. People on the Web show some tendency to speak of physical science as the construction of "mathematical models". This is a characterization inviting emendation into a still less flattering phrase - "mere mathematical models".
A Nixon-era USA television programme called "Rowan & Martin's Laugh-In" featured a Black comedian, "Flip Wilson", who at one or more points appeared on the screen in drag. "The devil made me put on this dress," said the loudspeaker voice, in possibly Southern cadences: "Ah said, yew stay away from ME, devil..." A parallel Satanic influence compels me to recount here my little story of mathematical modelling - scant though the value of my anecdote is in illuminating my wider theme, Choice of Tactics for Conserving Appreciation of Scientific Method. Readers concerned to save time can safely skip down as far as the phrase "very nice lady sitting beside me", knowing that they will thereby miss nothing of substance.
The most demanding and frightening, maths course I have taken was not in third year at all, but at second-year level - the University of Toronto 1991-1992 MAT257. MAT257 was a full-year presentation of multivariate real analysis. It culminated, I guess, in a presentation of Green's, Stokes's, and Gauss's theorems as special cases of one single grand theorem involving, I guess with respect to orientable manifolds immersed in real Euclidean spaces of arbitrarily high finite dimension, "differential forms".
This grim rite de passage, in comparison with which rites of adolescent passage in the sub-Saharan interior or in the Papua New Guinea highlands must rank as trifling inconveniences, was in the hands of an expositor of the first rank, the then-young Prof. E. Bierstone. I think professionals will see the gravity of his proceedings as soon as I cite his textbook: it was, alas, Spivak's Calculus on Manifolds.
I propose as a unit for measuring Academic Course Difficulty the "bier", named in my lecturer's honour. MAT257 attained in 1991-1992 a level of exactly 1 bier. Normally, courses are of a difficulty measured in nanobiers, or at worst in tens of millibiers.
Prof. Bierstone, while diligent and exact, was perhaps occasionally a little humourless. At a time at which we were pondering, among other things, the topological concept of an "open covering", Prof. Bierstone found himself having to make one of his infrequent administrative announcements. A competition, he said, was being organized, for such-and-such a venue and date, providing students in the Department the chance to test their skills in "mathematical modelling". We all had the same thought - even middle-aged I, whose hormones can no longer have been raging. Prof. Bierstone asked, in genuine puzzlement, why everyone was smiling or giggling, what everyone was thinking of.
I am in retrospect grateful that I managed to bite my tongue, avoiding the temptation to murmur to the very nice lady sitting beside me, or to say even more loudly - the temptation was there - "open coverings".
Mathematical models, like the observe-hypothesize-deduce-test schema, have their limited place.
In meteorology, it is normal to make numerical models, tiny grid square-or-cube by tiny grid square-or-cube, of the regional or global atmosphere. In such models, the supercomputing cluster - in the case of Canada, I gather the national machine resides in the Montréal suburb of Dorval - steps in some finite-differences way through the tens or hundreds or thousands of coupled differential equations. Such "numerical models" do perhaps in a sense explain something about the weather.
And in a more exalted setting, they do explain something about the inner structure of the Sun. In the solar case, the prof forms a much smaller set of equations, governing spatial variations in temperature and pressure over successively deeper solar layers, and uses her or his computer to work out the temperatures and pressures at great depths from plausible conjectures in nuclear physics, along with a knowledge of the Sun's size, mass, and observable skins - notably, from a knowledge of the Sun's photospheric spectral energy distribution, attainable observationally. - I write here "her or his computer" to mark my suspicion that a lone 1990s Intel 486 DX chip, as opposed to a contemporary Dorval-based Environment Canada supercomputing cluster, would be up to this particular numerical astrophysical task.
These two resorts to explanation through mathematical modelling are rather plebian. There may be some temptation in some quarters to say that this is as good as mathematics ever does get.
****
A perpetual theme in post-mediaeval philosophy is the recourse to what we might call "Nothing-Buttery".
The supposition of a necessitating causal nexus between events is (it was asserted by 18th-century David Hume) "nothing but" a projection onto the outside world of an inner feeling of confident expectation, this feeling being itself a sort of Pavlovian reflex. - If expounded carefully, the position at any rate escapes the reasonably obvious, undergraduate-level, objection, "Is there a necessitating causal nexus, then, within the mind, necessitating the emergence of the Pavlovian reflex after the projection-prone mind has undergone sufficient observational conditioning?" Even within the realm of the mind, Hume's duly careful expositor will say, putative causal necessities are a mere mythologizing projection.
Mathematics (it was asserted in the 1930s Vienna Circle, contrary to the then-recent adverse experiences of logicians Frege, Russell, and Whitehead) is "nothing but" an edifice of logical necessities.
The thinking familiar to humans is (so the eminent mathematical logician, and wartime codebreaker, Alan Turing (1912-1954) asserted in this 1950 paper, "Computing Machinery and Intelligence") "nothing but" the ability of a physical system, such as a machine, to pass an appropriate simulation test at the hands of human interrogators.
Again, we have in ethics the suggestion that moral duties are "nothing but" arrangements enforced by the positive law of a given society, or alternatively "nothing but" commands residing in the mind of a deity. (A more traditionally Catholic position, opposed to moral Nothing-Buttery in its theological variant, is that if, say, God enjoins some particular form of altruism, then God is enjoining it because it is right. It is not that the thing is right merely because God enjoins it; God is indeed, on the traditional Catholic approach, no more free to say "Evil, be thou my good" than God is free to defy the topology of the Moebius strip. One then proceeds to argue, along lines that are not particularly problematic, that these limitations on God are consistent with divine omnipotence, rightly understood - that omnipotence demands ability to select, as possible feats, only from the domain of feats coherently describable. C.S.Lewis would say that while God "can do anything", there is literally "no such thing" as dissecting the Moebius strip along its median into two separate strips.)
Indeed one might imagine a reductionist, or nothing-but, position in the field of natural essences that I noted here on 2016-06-14. On this possible philosophical position, there are no intrinsically natural ways of carving up the world. To return to my past example: we regard these two maples as belonging to the same "natural kind", taxonomically Acer saccharum, and this third maple as belonging to a different "natural kind", Acer rubrum. But (so the position goes) there is really no right or wrong way to classify things - no deep reason why the second and third trees could not be assigned the same classification, and the first one some different classification. Classifications, it is in a reductionist spirit suggested, are made for reasons of mere practical convenience, as municipal borders might by arbitrary fiat be drawn and redrawn, or currency exchange rates shifted down and up.
Without here delving further into Nothing-Buttery, I will take it that my readers are willing to ponder in a provisional way a philosophical position which opposes some forms of Nothing-Buttery. I invite my readers simply to take this position on provisionally, and to see, in a spirit of open-minded exploration, where it takes us. So let us in particular suppose, provisionally, that mathematics in physical science is not confined to mere "mathematical modelling". If Nothing-Buttery is rejected here, then we can see where our conservationist priorities must lie as our Dark Ages deepen.
****
A moment's reflection shows how tricky the conservationist task is.
In part, admittedly, inculcating an appreciation of scientific method, on its explanatory-mathematics side, can come from appropriately deep studies of work already done. Here there is little that is tricky. In electromagnetic theory one simply works, at second-year level, from some such author as E.M. Purcell - the clear, inventive expositor who in his earlier life shared with Felix Bloch a Nobel Prize for MRI-related work, and additionally in earlier life detected the 21-centimetre emission in radio astronomy. (It is true that it might prove best to take Purcell's latest, revised, posthumous edition, in which the units are SI rather than c.g.s.. Additionally, it might prove best to use Daniel Fleisch's recent Student's Guide to Maxwell's Equations, or something of this kind, as a lead-in.) And I gather from the pages of Daniel Fleisch and the like that no matter what profs in my early-1990s day may or may not have done at the University of Toronto, it is at any rate eventually normal - if not in third year then either in fourth year or in grad school - to work from J.D. Jackson's Classical Electrodynamics.
There will be lots of problem sets, of some rather standard character, and of course the prof will insist that his or her pupils battle diligently with the problem sets before imploring classmates or Teaching Assistants for hints.
So what is tricky?
What is tricky is that if we stop here, we have covered, so to speak, just two-thirds of the necessary terrain. With the remaining, mission-critical, third left uncovered, we risk falling into a kind of scholasticism. It would be a scholasticism paralleling, at a superficially impressive level of mathematical erudition, the mediaeval-scholastic ossification of Aristotelian biology. We have to add, somehow, the spark of something like original investigation, even as we work within the classics.
The Michelson-Morley experiment, as an educational tool, was much discussed over the week following 2016-02-17 at John Michael Greer's http://thearchdruidreport.blogspot.ca/2016/02/retrotopia-back-to-what-worked.html. I felt uncomfortable at the time, as one of Mr Greer's blog commenters. Here, I felt (though I did not say it too bluntly, or did not say it at all) we have a sterile recitation of authorities, worthy of the Sorbonne of, say, 1395, dealing dogmatically with, say, Aristotle's Historia animalium.
I did for my part try to propose for the classroom some lines of experimentation that would stimulate original conceptual work, as Michelson-Morley in my suspicion does not. In addition to commenting at that blog in the days following 2016-02-17 on Michelson-Morley itself, I suggested for Mr Greer's potential Looming-Dark-Ages classroom the following:
- An astronomical determination of the North direction. (Here I stressed that the superficially convenient bright star Polaris has only an accidental connection with the concept of North, being not in fact exactly at the North celestial pole. I suggested some appropriate questions for pupils who might be working this problem in the day rather than at night: how, for instance, do we get a properly planar and properly horizontal surface as we prepare to start measuring those plumb-line shadows?)
- A rerun of Cavendish's torsion-balance determination of the proportionality constant in the law of universal gravitation. (The law is that the strength of gravitational attraction between point masses is inversely proportional to the square of their separation. With the proportionality constant determined, and with the Earth's radius known, and with the gravitational acceleration of falling bodies measured near the Earth's surface, it is possible - I perhaps neglected to point this out on Mr Greer's blog - to achieve the seemingly miraculous, namely a determination of the mass of the Earth, as a number of, e.g., kilograms. The mass proves greater than the mass of an Earth-sized sphere of mere rock, making this result a potential portal into still further science.)
- An inexpensive classroom or home-lab investigation suggested by Richard Feynman (1918-1988; he originated quantum electrodynamics), on which one determines, with graduated cylinder, medicine dropper, glass tray, and square-ruled paper the thickness of an oil slick. I additionally pointed out some helpful lines of tutorial discussion once this Feynman assignment is coupled with the (also inexpensive) Young double-slit experiment, taken as a method of determining the wavelengths of variously coloured lights.
- An investigation, in the concept of Coriolis pseudo-forces, into the discrepancy between cloud directions of movement and weather-vane-determined wind directions.
The fact that I failed can be inferred from the character of the contemporaneous discussion, duly archived at http://thearchdruidreport.blogspot.ca/2016/02/retrotopia-back-to-what-worked.html. Although the various discussants addressed Mr Greer's ideas, or mine, or both, nobody took my various offerings as an occasion for conceptual probing in physics.
In hindsight, I realize now that I should not, in my battle against impending neomediaeval scientific sterility, have strayed far from Einstein and Maxwell. I should have introduced into Mr Greer's envisaged classroom just one thing - properly anchored, as indeed Michelson-Morley is, in the Maxwell-Einstein classics, and yet adequately subtle. This one thing illustrates, by way of example - I see no good way of working except through example - an approach to the delicate side of the conservation task. It shows what is needed if the ever-so-important last third of the terrain, going beyond conventional problem-set assignments, is to be covered.
To avoid making mistakes, I here adopt a cowardly literary expedient, posing Socratic questions instead of making assertions. But the question is in any case to be preferred in tutorial work to the assertion:
First Question: Is it true that (as stated in popularizing, pre-university, treatments of electromagnetism) "a voltage is induced in a conductor moving so as to cut magnetic lines of flux"? A uniform magnetic field of rather large spatial extent, sustained by (say) a pair of Helmholtz coils of rather generous radius, carrying direct currents, runs parallel to the lab floor. A tiny copper ring, its plane perpendicular to the field, moves downward through the field. Is there some electric-current-driving induced electric field all the way around the ring, or not?
Variant on First Question: Can the presence or, as it might perhaps be, the absence of electric current in this situation be explained in terms of Lorentz forces on individual charge carriers? Let the ring be deformed into a tiny rectangle ABCD, with sides AB and CD parallel to the field, and with sides BC and DA consequently perpendicular to the field. What Lorentz forces (consider directions where appropriate) are experienced by the mobile electrons in the copper in each of these four respective sides?
Rider to Variant on First Question: What, in terms of Maxwell's equations, can be said about - to use my 2016-06-07 language - "Magnetic Illumination" of capping surfaces for the non-self-intersecting loop which is the rectangle ABCD? (Is the "Magnetic Illumination" constant or varying?) What follows regarding presence or absence of an induced electric field-all-the-way-around-the-rectangle?
Second Question: What happens when the rectangle ABCD, while kept perpendicular to the immersing magnetic field, has an axle, parallel to the field, attached to some point on one of its sides, with this axle now caused to spin? (The rectangle in this Gedankenexperiment is moving through the field in the manner of a lawnmower half-blade cutting grass stalks.) What should now be said, in a Maxwell context, regarding variation or failure-to-vary of "Magnetic Illumination" aggregated over capping surface? What is now the case regarding electric fields-all-the-way-around-the-rectangle and the electric currents which they may putatively drive?
Third Question: What happens when the whole surface swept out by the axle-driven rectangle ABCD is replaced by a solid copper disk (with the axle consequently at its centre, driving the disk like the disk sander in a workshop)? What, in terms of current-driving electric fields and consequent electric currents, is observed when conducting brushes are applied, as at Figure One in https://en.wikipedia.org/wiki/Faraday_paradox? Do the various closed non-self-intersecting loops that can be drawn on this disk refute the applicable Maxwell law for electric fields?
Here we reach, through just a short line of questioning, depths which I have not yet mastered, and yet which I and all other students of electromagnetics (even humble radiotelegraph designers in the upcoming Dark Ages, for whose ultimate benefit I hope in future to be writing) will have on pain of disgrace to master.
I bet full mastery will involve close attention to questions regarding choice of reference frame - rest frame of lab? or, rather, rest frame of such-and-such a path segment in the closed non-self-intersecting loop?
I bet, further, that full mastery will involve deploying mathematics of explanatory power, with tensors as opposed to mere vectors, in a duly Einsteinean Special-Relativity setting. Some light is almost certainly shed by the deep Bell Labs thinker (he really is, in real life, called "Dr John Denker") who has published at https://www.av8n.com/physics/faraday-puzzle.htm. But I dare not study his essay before I have done my own "Faraday's Paradox" or "Faraday's Puzzle" thinking.
Finally, I bet, or rather I already know, that full treatment of this "Faraday Paradox" puzzle will involve not merely maths, but supporting lab work, at any rate to confirm the correctness of the various mathematical deductions. To my annoyance, I fail to retreive today, either from my generally well organized Scotland Yard notes or via Google, the relevant Ottawa-based home experimenter in this field. But the abundance of other laboratory material is today suggested by, e.g., a quick Google Images search under the string homopolar generator experiments.
[Coming next week, in the upload of UTC=20160628T0001Z/20160628T0401Z: A discussion of some nuts-and-bolts in conservation of scientific-method knowledge, quite likely with reference to the Vatican Observatory Research Group and other aspects of Catholic scientific life. It is rather likely that next week's upload will conclude this essay. In a separate essay, at some later point, I shall have to discuss the practicalities of work at my own desk, involving such things as the erasable coloured pencils and the just-cited - and just-now-disappointing - "Scotland Yard" files.]
Tuesday 14 June 2016
Toomas Karmo (Part C): Is Science Doomed?
Quality assessment:
[CAUTION: A bug in the blogger
software has shown a propensity to insert inappropriate whitespace at
some late points in some of my posted essays. If a screen seems to end
in empty space, keep scrolling down. The end of the posting is not
reached until the usual blogger "Posted by Toomas (Tom) Karmo at"
appears.]
In my admittedly ill-informed view, some suggestion that not all necessities in physical science are straightforwardly definitional emerges not only from Kripkean necessities anchored in "substantial essences", but additionally from the failure of properly strenuous efforts made, from Frege onward, to exhibit mathematics as a collection of merely definitional truths.
The programme of Frege, soon taken up also by the young Bertrand Russell (1872-1970), was to exhibit the full sweep of physics-pertinent mathematics as an edifice of tautologies, each having the metaphysically reassuring emptiness of the only-trivially-necessary "All triangles have three sides." It is perhaps fair to say that the Frege-Russell programme failed, and that the metaphysically troubling spectre of necessities-which-are-not-merely-definitional survived.
Frege's offered edifice was found by Russell to be internally inconsistent. Russell, writing to a length of three fat volumes with Alfred North Whitehead (1861-1947), claimed to restore consistency through a revision of Frege's theory, dividing the possible properties of individuals into a hierarchy of logical "Types".
I gather that to recover, as the pinnacle of the imposing edifice, the corpus of physics-pertinent mathematics, is to proceed from purely logical constructions right out to Peano's axioms for the arithmetical structure of the set {0, 1, 2, ... }. From this point onward, I gather, the well established results of other workers kick in, as when one defines the negative numbers; and then the rationals; and then the reals; and then the derivatives and integrals of the more suitably housebroken univariate real functions; and so on, onward and upward, through Div and Grad and Curl in multivariate real calculus; eventually also with physics-pertinent constructions, including calculus, in the complex-number plane.
To recover the corpus of physics-pertinent mathematics, Russell found it necessary to introduce a new postulate, the "Axiom of Reducibility". His Axiom, in my admittedly ill-informed view, is not plausibly called a principle of logic, but a piece of substantive mathematics.
If, now, physics-pertinent mathematics stubbornly retains (in light of Frege's and Russell-Whitehead's adverse experiences) a necessity deeper than the merely definitional, might the same not be true (I ask) for physical laws themselves, or at least for some aspects of those laws - if not, for instance, for the value of Coulomb's proportionality constant in some selected system of physical units, then at least for his so-tidy value "2" for that denominator exponent?
I suggested that Coulomb's exponent might be anchored in necessities of geometry. It will be objected that geometry was itself found by Riemann and Lobachevsky to be infected with contingency, in researches ultimately calling into question even the inevitability of classically accepted formulae for areas of surfaces. Indeed (it will be objected) the very question whether physical space is Euclidean or non-Euclidean nowadays gets one answer near a massive body and a different answer when massive bodies are removed.
To this objection, I reply that if I am wrong in my suggestion about Coloumb, nevertheless - whatever may be the case with the contingency, in General Relativity, of the local curvature of space - possibilities for deeper necessities remain. For example, it as far as I have read necessary that when that closed non-self-intersecting single-sided single-edged loop which is a Moebius strip is cut with scissors along its median, it does not fall apart into two closed non-self-intersecting loops. It instead opens up, and I imagine cannot be prevented even by God from opening up, into a single, rather vigorously twisted, closed non-self-intersecting loop.
And the mere fact that space (as opposed to Minkowski spacetime) has three dimensions might be a necessity in its own right. At any one instant - we keep time out of this, staying purely spatial - at any point P in space, there can be at-least-and-at-most three vanishingly thin mutually perpendicular metre sticks with their zero ends touching at P. Although we can describe, in the framework of linear algebra, spaces of 4, 5, 6, ... dimensions, our own physical space (however locally varying its Euclid-defying curvature) seems resolutely 3-dimensional. This limitation does not appear a mere contingent matter.
Or is even this wrong? Has String Theory, or some similar physics formalism, shown a way to lift the limitation to three spatial dimensions? All I really dare say here is that the questions are weighty.
Whatever the precise mixture of contingency and necessity in the world of physical laws, it is at any rate evident that the laws constitute the core of physical science, with such prima facie contingencies as the value of the Hubble constant tending to lie more on the periphery.
We can now see where conservation priorities must lie as we decide, so to speak, how far behind the breached Mannerheim Line our forces shall retreat.
[To be continued, and perhaps concluded, in the upload of UTC=20160621T0001Z/20160621T0401Z. As I have outlined the writing, I am to finish this essay with some remarks on the organization of science research and the organization of science education. My envisaged additional remarks, on the practicalities of my own private programme of work in mathematics or physics - the resort to three colours of pencil; the MI-6-worthy filing system; the principle of reading more than one author on any single given topic; and above all the precept "Close the book, work it out alone, then open the book" - is best deferred to some subsequent essay, at some either near or distant point in 2016.]
On the
5-point scale current in Estonia, and surely in nearby nations, and
familiar to observers of the academic arrangements of the late,
unlamented, Union of Soviet Socialist Republics (applying the easy and
lax standards Kmo deploys in his grubby imaginary "Aleksandr
Stepanovitsh Popovi nimeline sangarliku raadio instituut" (the
"Alexandr Stepanovitch Popov Institute of Heroic Radio") and his grubby
imaginary "Nikolai Ivanovitsh Lobatshevski nimeline sotsalitsliku
matemaatika instituut" (the "Nicolai Ivanovich Lobachevsky Institute of
Socialist Mathematics") - where, on the lax and easy grading philosophy
of the twin Institutes, 1/5 is "epic fail", 2/5 is "failure not so
disastrous as to be epic", 3'5 is "mediocre pass", 4.5 is "good", and
5/5 is "excellent"): 4/5. Justification: There was enough time to
develop the relevant points to reasonable length.
Revision history:
- UTC=20160615T1624Z/version 1.1.0: Kmo added several paragraphs, trying to do better justice to logician-philosopher Saul Kripke's "Naming and Necessity", and to bring out more clearly the idea of physical science as an investigation of "substantial essences" (and he reserved the right to upload, over the coming few days, without formal documentation in this revision history, nonsubstantive revisions, as version 1.1.1, 1.1.2, ... ).
- UTC=20160614T0047Z/version 1.0.0: Kmo finished an interrupted uploaded of base version (and planned to upload in the ensuing four-hour
interval, without formal documentation in this revision history,
nonsubstantive revisions, as versions 1.0.1, 1.0.2, ... .
4. The Coming Dark-Ages Triage of Physical Science
The prospects for physical science, in our ongoing civilizational decline, resemble the prospects for a republic on the brink of destruction by Stalin.
What may we have to face?
Over the next four decades or so, at any rate assuming a continuation of trends and conditions from the last four decades (and, in particular, assuming no widescale thermonuclear war), we face no apocalypse. We may expect the standards of high schools - relevant to science as a source of supply for university admissions offices - to sink still lower. We may expect the current scandals, notably around inadequately refereed journals, to continue. This is a development which will make the already rather cynical public appraisal of scientists increasingly like the current cynical public appraisal of those social outcasts who are the lawyers, the politicians, and the property developers. With this ongoing decline in public esteem for science, eloquently analyzed by John Michael Greet on 2014-11-26 at http://thearchdruidreport.blogspot.ca/2014/11/dark-age-america-suicide-of-science.html, we may expect a concomitant contraction in public funding.
And I for one would expect public superstition to rise correspondingly, as with the gullible people already making it commercially realistic for "psychics" to advertise their services here in Richmond Hill, Ontario.
But I am interested in the present essay in the long term, over the next two or three or four centuries. Our best understanding of that long term suggests a contraction in the available supply of fossil fuels, and yet also (because we are at present abundantly burning the abundant supplies we have, rendering them temporary) a change in climate. What can the social environment of science be like in a world in which even the Monument and Bank Tube stations, perhaps even some or all floors of the present Bank of England, are submerged at high tide?
We may well imagine that at this stage governments and literacy, as we now know them, will have faded.
In place of Number Ten Downing Street, the Oval Office, and the like, we may reasonably predict warlords. In place of the British Library, we may reasonably predict what - total illiteracy? I would envisage, rather, a world in which the powerful brandish their literacy as a badge of authority, keeping finely bound classics on their manor-house shelves, and turning their nasty little surviving universities (their Yales, their Oxfords) into socially exclusive social-networking tools, into which only they and their special allies penetrate easily. The serfs would in this scenario be supplied with only as much education as might be needed for them to meet manorial needs.
If, in that impending Dark Age, serfs can be distracted with forms of pseudo-education, in which they take degrees in "Media Studies" and the like (rather than learning such potentially empowering things as Roman law, Church history, modern languages, and differential equations), then all the better. But in that warm, humid, impoverished, ill-lit world, pseudo-educating the serfs will perhaps prove beyond the economic means of most jurisdictions.
In an era in which education is radically de-democratized, there will be a demand less for science than for engineering. Here the more able of the serfs will get something. Any warlord of administrative ability will appreciate, for example, the value of aerial reconnaissance - by drones with cameras, if sufficient engineering skills have survived, but otherwise through balloon techniques familiar to Generals Grant and Lee.
And any warlord worth his salt will appreciate the value of communications. We may well imagine things stabilizing at a level appreciably higher than the merely Victorian. Transistors - so dependent on pure materials and clean-room technique - may perhaps be gone. But what is likely to remain, as an empowering technology in a violent world, is the suitcase-sized Morse transmitter, its little "peanut valves" glowing gently on the strength of chemical batteries. Such a set would be skilfully operated, even skilfully maintained, in the field by some private or lieutenant, born in some trim little cottage on the manorial estates, and trained in the relevant engineering at his lord's expense.
So our question pertains to physical science, not to engineering. And our question is not whether physical science is doomed in the next four decades. Rather, the question is whether physical science is doomed over the long term - say, over the next four centuries.
****
Republics on the brink of destruction by Stalin come in different varieties. At one extreme in the autumn of 1939 is Estonia. At a different extreme is Finland.
In that autumn, Estonia found itself incapable of conserving anything. With Moscow's ultimatum in hand, the careful defensive preparations of the entire 1920-1939 period were rendered irrelevant. (A deal had been made with the UK, I think early in 1939, to upgrade a part of the air force to state-of-the-art Spitfires; bridges had long been built with cavities in their piers, to facilitate quick destruction by Estonian land forces at the instant of retreat; military radio is likely to have been solidly engineered, since the civilian telephone service, equipped with shortwave, was itself offering duly wealthy civilian subscribers the operator placing of long-distance calls to Japan and Malaya; and there was a volunteer "Defence League" over and above the formal armed services. All this, and much else, had to be set aside, instantly.) It was clear to the government of the day, after deliberations in Tallinn lasting an hour or so, at any rate not for even as long as two hours, that just one single outcome was possible in the event of hostilities with Moscow.
Finland, to whom Stalin handed a similar ultimatum that autumn, likewise made a calculation correctly fitting its circumstances. Its circumstances, however, were different and less bleak. In Finland's case, Stalin's ultimatum was rejected, with resistance over the next few months successfully mounted - and this even though, in the terrifying event, Karelia was lost, and (contrary to the rational expectations of non-Finnish analysts) the Mannerheim Line was breached.
The Republic of Physical Science does not, over the long term, confront annihilation in the manner of 1939 Estonia. Neither, on the other hand, does it rejoice in the prospect of survival in the robust manner of post-1939 Finland. Our task here is to locate the truth which lies somewhere between these two extremes. Much will have to be lost - not just (to pursue the Finnish comparison) Viipuri and its Karelian hinterland, but perhaps Turku and Helsinki. And yet something - some austere little Oulu, some harsh little Rovaniemi, so far north that the December sun scarcely rises - can be kept going.
Where must our priorities lie?
Where must our priorities lie?
****
Physical science is in part an investigation of matters that are prima facie contingent. What, for example, is the value of the Hubble Constant, which yields the recessional speed, say in our galaxy-centre rest frame, of an external galaxy at some given distance? (On looking this up today in you-know-what, I found a surprisingly large recent recalibration: apparently, although the ESA Planck Mission published 67.80±0.77 km/s per megaparsec on 2013-03-21, the Hubble Space Telescope people published 73.00±1.75 km/s per megaparsec on 2016-05-17: https://en.wikipedia.org/wiki/Hubble's_law#Observed_values .)
I presume that as far as any of the profs know, there is no reason for calling the modal status of the Hubble Constant value anything but "contingent". In some state of affairs logically consistent with all the known fundamental physical laws, the Constant, I presume, has one value. In some other states of affairs logically consistent with all the known fundamental physical laws, the Constant, I presume, has a different value. And I presume it is even in some sense possible that the "Constant" assumes different values at different stages in the history of the cosmos.
A similar, more humble, example of a radically contingent matter is the present pressure (say, in newtons per square metre) at the core of our own Sun.
A more important part of physical science, however, looks not into contingent particulars, such as the pair just cited, but into universal principles.
Before developing my argument regarding conservation priorities in a new scientific Dark Age, I want to highlight the special status of such principles. I wish to do so by exploring a question too little discussed - the question of the modal status of laws. Are they, like particular facts, contingent? Or do they possess, at least in some of their aspects, some relevant element of necessity?
Consider the principle that the acceleration experienced by a body in an inertial frame is directly proportional to the force on the body times the inertial mass of the body. If forces, masses, and accelerations are measured in the usual "SI" system of units, or again in the alternative "cgs" system that has been prevalent in astrophysics, this becomes the familiar F= ma, i.e., F = kma with constant k becoming the purely numeric quantity 1.
I had the good luck to have, as a tutor in first year, the University of Toronto low-temperature experimentalist Cindy Krysak. She was not satisfied with us, her tutorial group, until we were willing to chant, loudly, in the manner of a football crowd, when she asked "So what's the first thing to remember on your midterm?", "F EQUALS M A! F EQUALS M A!"
What is here contingent, and what is here a matter of definition? It is no contingent matter that all triangles have three sides. A four-sided polygon would by definition not be a triangle. Analogously, we may well feel, something which was not related to force and acceleration as a factor-in-a-proportionality, as obediently chanted by Cindy's mob of frosh, would no longer qualify as an "inertial mass".
The importance of laws in the edifice of physical science becomes all the more striking when we realize, upon further reflection, that the concept of mass conceals conceptual depths, ultimately linked to General Relativity or cognate disciplines. An elementary Newtonian study of astrophysics, as in orbital mechanics, reveals the need to introduce a concept correlative with inertial mass, "gravitational mass". But then arises a question pressed by the General Relativity gurus: why do the two quantities, introduced to physics in such observationally disparate ways, seem always equal?
Or consider, again, Coulomb's electrostatic law, according to which the attractive or repulsive force between two charges (each of negligible spatial extent) is inversely proportional to the square of their separation. What is here contingent, and what is here necessary? Without having pondered this beyond a second-year level, I would suspect on the one hand that the constant of proportionality (once we fix on, say, SI units) is contingent, and that on the other hand some deep necessity, perhaps of a geometrical origin, attaches to the "2" exponent. Perhaps the "2" is at some deep level related to the fact that the area of a sphere increases as the square of its radius. For this fact about areas has the consequence that the illumination-per-square-metre of a sphere enclosing a lamp, or analogously the electrical "Illumination"-per-square-metre of a sphere enclosing a charge, is inversely proportional to the square of the radius. Perhaps to imagine that the exponent is not 2 but, say, 2.000001 is to imagine geometry as being something other than what it in physical space inevitably is.
Admittedly, the suspicion of a geometrical necessity does not release us from the obligation to do experiments, as a check on the correctness of whatever a priori reasoning we may construct. On today consulting my copy of that thoroughly laboratory-anchored exposition, at second-year level, which is W.J. Duffin's Electricity and Magnetism (McGraw-Hill, fourth edition, 1990), I find references to several workers, from Cavendish in 1772 (in an investigation not published until 1879) up to Williams-Faller-Hill (said by Duffin to be in Phys. Rev. Letters 26 (1971)).
If physical science, on its observatory-dome side, finds reason for thinking that "laws" change from one part of the cosmos to another, then this will only mean that the supposed laws are not true laws - that either (a) there are no laws at all (beyond, that is, the minimal, negative law that There Are No Non-Minimal Laws) or (b) the local "laws" are locally varying instances of some underlying universal principle which itself qualifies as a law.
It seems to me that up to 1990, when I left professional academic philosophy, there had been insufficient discussion of the modal status of physical laws. A promising beginning had, indeed, been made by Saul Kripke (1940-) at Princeton, who had pointed out the noncontingent character of such propositions as "Gold has atomic number 79." Any element with a different number of nucleus protons, Kripke had remarked in his well-titled "Naming and Necsssity", could not be gold. If there is a possible world in which an element of atomic number 82 has the mass, ductility, conductivity, and colour and sheen, and so forth, of gold, then in this possible world it would be lead, not gold, which possessed the so-to-speak Golden Phenomenology.
Although it is in a sense true that "Gold has atomic number 79" is a definitional necessity, one hesitates to say here (as one unblushingly says for "Every triangle has three sides") that it is a definitional necessity of a banal kind - that it is a, so to speak, "mere" definitional necessity. I wrote incautiously in the 1.0.0, 1.0.1, 1.0.2, ... series of versions of this essay, calling Kripke's proposition "perhaps straightforwardly definitional". But the ancients and mediaevals, ignorant of the atomic nucleus, nevertheless succeeded in attaching a duly definite meaning to the term "gold". In the admittedly none-too-clear language of their Aristotelian tradition, we might say that they correctly meant the term "gold" to signify some "substantial essence" unknown to them, and explored only in modern times.
It might be further suggested that physical science is quite generally the study of "substantial essences", and that much remains to be done. What, for example, is a "substantial essence" of a Linnean species of tree, such as the red maple, Acer rubrum? Something will have to be said here about DNA.
The underlying conceptual problems here assume a certain - to me unfathomable - complexity when it is noted that the DNA can remain intact even in a dead specimen of Acer rubrum. Perhaps the full story of the "substantial essence" will involve some duly mathematical considerations of something like entropy flows. (I did at one point read a book with the promising title Entropy for Biologists, but cannot claim to have retained from it anything beyond television-and-magazine-level trivialities.)
A short remark which I published in a philosophical journal (Analysis 37 (1977), pp. 147-148, under the title "Disturbances") might - or might not - be helpful. I remarked that a living thing resembles a knot, in that just as a knot can migrate along a rope, so a living thing migrates through consignments of chemicals, progressively ingesting and excreting stuffs. As a knot is in a rope, so, I suggested, is a living thing "in" its constituent matter. I quoted in this context, as potentially useful, Aristotle's Metaphysics 1034a6 dictum that that the "form" of a human being is "in" his flesh and bones"; Aristotle's comparison of living tissues with flowing water at de Gen. et Corr. 321b24-25; and Aristotle's suggestion at Topics 127a3-8 that a wind is better defined as a movement in air than as air in movement. Perhaps substantial essences of living things involve "forms" - in some as-yet-unknown sense to be rendered precise through mathematical insights both into DNA and into entropy - migrating "through" stuffs?
One imagines that even the DNA story (leaving the mathematics of entropy aside for the moment) will bristle with subtleties. We have in DNA a strange approach of biology to formal computer science. But how mature, as a branch of mathematics (as opposed to the mere engineering of hardware, in the now-hoary 1940s-onward von Neumann/Harvard machine-architecture tradition) is today's computer science? And how well understood are the mathematical formalities of DNA? One does, as a layman, get the impression that while those parts of the double helix which encode proteins are at some level understood, unclarities abound in the remainder, and that some of the professionals are nowadays uneasy with the dismissive term "junk DNA".
****
Consider the principle that the acceleration experienced by a body in an inertial frame is directly proportional to the force on the body times the inertial mass of the body. If forces, masses, and accelerations are measured in the usual "SI" system of units, or again in the alternative "cgs" system that has been prevalent in astrophysics, this becomes the familiar F= ma, i.e., F = kma with constant k becoming the purely numeric quantity 1.
I had the good luck to have, as a tutor in first year, the University of Toronto low-temperature experimentalist Cindy Krysak. She was not satisfied with us, her tutorial group, until we were willing to chant, loudly, in the manner of a football crowd, when she asked "So what's the first thing to remember on your midterm?", "F EQUALS M A! F EQUALS M A!"
What is here contingent, and what is here a matter of definition? It is no contingent matter that all triangles have three sides. A four-sided polygon would by definition not be a triangle. Analogously, we may well feel, something which was not related to force and acceleration as a factor-in-a-proportionality, as obediently chanted by Cindy's mob of frosh, would no longer qualify as an "inertial mass".
The importance of laws in the edifice of physical science becomes all the more striking when we realize, upon further reflection, that the concept of mass conceals conceptual depths, ultimately linked to General Relativity or cognate disciplines. An elementary Newtonian study of astrophysics, as in orbital mechanics, reveals the need to introduce a concept correlative with inertial mass, "gravitational mass". But then arises a question pressed by the General Relativity gurus: why do the two quantities, introduced to physics in such observationally disparate ways, seem always equal?
Or consider, again, Coulomb's electrostatic law, according to which the attractive or repulsive force between two charges (each of negligible spatial extent) is inversely proportional to the square of their separation. What is here contingent, and what is here necessary? Without having pondered this beyond a second-year level, I would suspect on the one hand that the constant of proportionality (once we fix on, say, SI units) is contingent, and that on the other hand some deep necessity, perhaps of a geometrical origin, attaches to the "2" exponent. Perhaps the "2" is at some deep level related to the fact that the area of a sphere increases as the square of its radius. For this fact about areas has the consequence that the illumination-per-square-metre of a sphere enclosing a lamp, or analogously the electrical "Illumination"-per-square-metre of a sphere enclosing a charge, is inversely proportional to the square of the radius. Perhaps to imagine that the exponent is not 2 but, say, 2.000001 is to imagine geometry as being something other than what it in physical space inevitably is.
Admittedly, the suspicion of a geometrical necessity does not release us from the obligation to do experiments, as a check on the correctness of whatever a priori reasoning we may construct. On today consulting my copy of that thoroughly laboratory-anchored exposition, at second-year level, which is W.J. Duffin's Electricity and Magnetism (McGraw-Hill, fourth edition, 1990), I find references to several workers, from Cavendish in 1772 (in an investigation not published until 1879) up to Williams-Faller-Hill (said by Duffin to be in Phys. Rev. Letters 26 (1971)).
If physical science, on its observatory-dome side, finds reason for thinking that "laws" change from one part of the cosmos to another, then this will only mean that the supposed laws are not true laws - that either (a) there are no laws at all (beyond, that is, the minimal, negative law that There Are No Non-Minimal Laws) or (b) the local "laws" are locally varying instances of some underlying universal principle which itself qualifies as a law.
It seems to me that up to 1990, when I left professional academic philosophy, there had been insufficient discussion of the modal status of physical laws. A promising beginning had, indeed, been made by Saul Kripke (1940-) at Princeton, who had pointed out the noncontingent character of such propositions as "Gold has atomic number 79." Any element with a different number of nucleus protons, Kripke had remarked in his well-titled "Naming and Necsssity", could not be gold. If there is a possible world in which an element of atomic number 82 has the mass, ductility, conductivity, and colour and sheen, and so forth, of gold, then in this possible world it would be lead, not gold, which possessed the so-to-speak Golden Phenomenology.
Although it is in a sense true that "Gold has atomic number 79" is a definitional necessity, one hesitates to say here (as one unblushingly says for "Every triangle has three sides") that it is a definitional necessity of a banal kind - that it is a, so to speak, "mere" definitional necessity. I wrote incautiously in the 1.0.0, 1.0.1, 1.0.2, ... series of versions of this essay, calling Kripke's proposition "perhaps straightforwardly definitional". But the ancients and mediaevals, ignorant of the atomic nucleus, nevertheless succeeded in attaching a duly definite meaning to the term "gold". In the admittedly none-too-clear language of their Aristotelian tradition, we might say that they correctly meant the term "gold" to signify some "substantial essence" unknown to them, and explored only in modern times.
It might be further suggested that physical science is quite generally the study of "substantial essences", and that much remains to be done. What, for example, is a "substantial essence" of a Linnean species of tree, such as the red maple, Acer rubrum? Something will have to be said here about DNA.
The underlying conceptual problems here assume a certain - to me unfathomable - complexity when it is noted that the DNA can remain intact even in a dead specimen of Acer rubrum. Perhaps the full story of the "substantial essence" will involve some duly mathematical considerations of something like entropy flows. (I did at one point read a book with the promising title Entropy for Biologists, but cannot claim to have retained from it anything beyond television-and-magazine-level trivialities.)
A short remark which I published in a philosophical journal (Analysis 37 (1977), pp. 147-148, under the title "Disturbances") might - or might not - be helpful. I remarked that a living thing resembles a knot, in that just as a knot can migrate along a rope, so a living thing migrates through consignments of chemicals, progressively ingesting and excreting stuffs. As a knot is in a rope, so, I suggested, is a living thing "in" its constituent matter. I quoted in this context, as potentially useful, Aristotle's Metaphysics 1034a6 dictum that that the "form" of a human being is "in" his flesh and bones"; Aristotle's comparison of living tissues with flowing water at de Gen. et Corr. 321b24-25; and Aristotle's suggestion at Topics 127a3-8 that a wind is better defined as a movement in air than as air in movement. Perhaps substantial essences of living things involve "forms" - in some as-yet-unknown sense to be rendered precise through mathematical insights both into DNA and into entropy - migrating "through" stuffs?
One imagines that even the DNA story (leaving the mathematics of entropy aside for the moment) will bristle with subtleties. We have in DNA a strange approach of biology to formal computer science. But how mature, as a branch of mathematics (as opposed to the mere engineering of hardware, in the now-hoary 1940s-onward von Neumann/Harvard machine-architecture tradition) is today's computer science? And how well understood are the mathematical formalities of DNA? One does, as a layman, get the impression that while those parts of the double helix which encode proteins are at some level understood, unclarities abound in the remainder, and that some of the professionals are nowadays uneasy with the dismissive term "junk DNA".
The full story of the "substantial essence" of Acer rubrum might at any rate involve a vindication of taxonomists in the school of Linnaeus. Although ignorant of DNA and entropy flows, they insisted on some deep generic similarity, and on some concomitant deep specific difference, between, for instance, Acer rubrum and its cousin Acer saccharum (the sugar maple). Their procedure here recalls the still earlier study of chemical elements: the mediaevals insisted on some deep difference between gold and its look-alike pyrites, even while being unable to say what the difference consisted in.
****
In my admittedly ill-informed view, some suggestion that not all necessities in physical science are straightforwardly definitional emerges not only from Kripkean necessities anchored in "substantial essences", but additionally from the failure of properly strenuous efforts made, from Frege onward, to exhibit mathematics as a collection of merely definitional truths.
The programme of Frege, soon taken up also by the young Bertrand Russell (1872-1970), was to exhibit the full sweep of physics-pertinent mathematics as an edifice of tautologies, each having the metaphysically reassuring emptiness of the only-trivially-necessary "All triangles have three sides." It is perhaps fair to say that the Frege-Russell programme failed, and that the metaphysically troubling spectre of necessities-which-are-not-merely-definitional survived.
Frege's offered edifice was found by Russell to be internally inconsistent. Russell, writing to a length of three fat volumes with Alfred North Whitehead (1861-1947), claimed to restore consistency through a revision of Frege's theory, dividing the possible properties of individuals into a hierarchy of logical "Types".
I gather that to recover, as the pinnacle of the imposing edifice, the corpus of physics-pertinent mathematics, is to proceed from purely logical constructions right out to Peano's axioms for the arithmetical structure of the set {0, 1, 2, ... }. From this point onward, I gather, the well established results of other workers kick in, as when one defines the negative numbers; and then the rationals; and then the reals; and then the derivatives and integrals of the more suitably housebroken univariate real functions; and so on, onward and upward, through Div and Grad and Curl in multivariate real calculus; eventually also with physics-pertinent constructions, including calculus, in the complex-number plane.
To recover the corpus of physics-pertinent mathematics, Russell found it necessary to introduce a new postulate, the "Axiom of Reducibility". His Axiom, in my admittedly ill-informed view, is not plausibly called a principle of logic, but a piece of substantive mathematics.
If, now, physics-pertinent mathematics stubbornly retains (in light of Frege's and Russell-Whitehead's adverse experiences) a necessity deeper than the merely definitional, might the same not be true (I ask) for physical laws themselves, or at least for some aspects of those laws - if not, for instance, for the value of Coulomb's proportionality constant in some selected system of physical units, then at least for his so-tidy value "2" for that denominator exponent?
I suggested that Coulomb's exponent might be anchored in necessities of geometry. It will be objected that geometry was itself found by Riemann and Lobachevsky to be infected with contingency, in researches ultimately calling into question even the inevitability of classically accepted formulae for areas of surfaces. Indeed (it will be objected) the very question whether physical space is Euclidean or non-Euclidean nowadays gets one answer near a massive body and a different answer when massive bodies are removed.
To this objection, I reply that if I am wrong in my suggestion about Coloumb, nevertheless - whatever may be the case with the contingency, in General Relativity, of the local curvature of space - possibilities for deeper necessities remain. For example, it as far as I have read necessary that when that closed non-self-intersecting single-sided single-edged loop which is a Moebius strip is cut with scissors along its median, it does not fall apart into two closed non-self-intersecting loops. It instead opens up, and I imagine cannot be prevented even by God from opening up, into a single, rather vigorously twisted, closed non-self-intersecting loop.
And the mere fact that space (as opposed to Minkowski spacetime) has three dimensions might be a necessity in its own right. At any one instant - we keep time out of this, staying purely spatial - at any point P in space, there can be at-least-and-at-most three vanishingly thin mutually perpendicular metre sticks with their zero ends touching at P. Although we can describe, in the framework of linear algebra, spaces of 4, 5, 6, ... dimensions, our own physical space (however locally varying its Euclid-defying curvature) seems resolutely 3-dimensional. This limitation does not appear a mere contingent matter.
Or is even this wrong? Has String Theory, or some similar physics formalism, shown a way to lift the limitation to three spatial dimensions? All I really dare say here is that the questions are weighty.
Whatever the precise mixture of contingency and necessity in the world of physical laws, it is at any rate evident that the laws constitute the core of physical science, with such prima facie contingencies as the value of the Hubble constant tending to lie more on the periphery.
****
We can now see where conservation priorities must lie as we decide, so to speak, how far behind the breached Mannerheim Line our forces shall retreat.
[To be continued, and perhaps concluded, in the upload of UTC=20160621T0001Z/20160621T0401Z. As I have outlined the writing, I am to finish this essay with some remarks on the organization of science research and the organization of science education. My envisaged additional remarks, on the practicalities of my own private programme of work in mathematics or physics - the resort to three colours of pencil; the MI-6-worthy filing system; the principle of reading more than one author on any single given topic; and above all the precept "Close the book, work it out alone, then open the book" - is best deferred to some subsequent essay, at some either near or distant point in 2016.]
Subscribe to:
Posts (Atom)